WhatsApp memes with hidden beauty!

by

Introduction

I love internet memes. They provide a funny way to convey a message or an idea. As a professor, I cherish all didactic mechanisms and anything that easy the acquisition or fixation of ideias and concepts. In fact, I find memes to be a very useful didactic tool. I might make a post only about didactic memes in the future. Right now I want to talk about one specific meme that, although not didactic at first glance, hides a cool and rather formal math concept without most people realising it (except for the author, I bet!).

The fun…

So, here we go. This is the meme. Maybe you already received it via WhatsApp (I did %-).

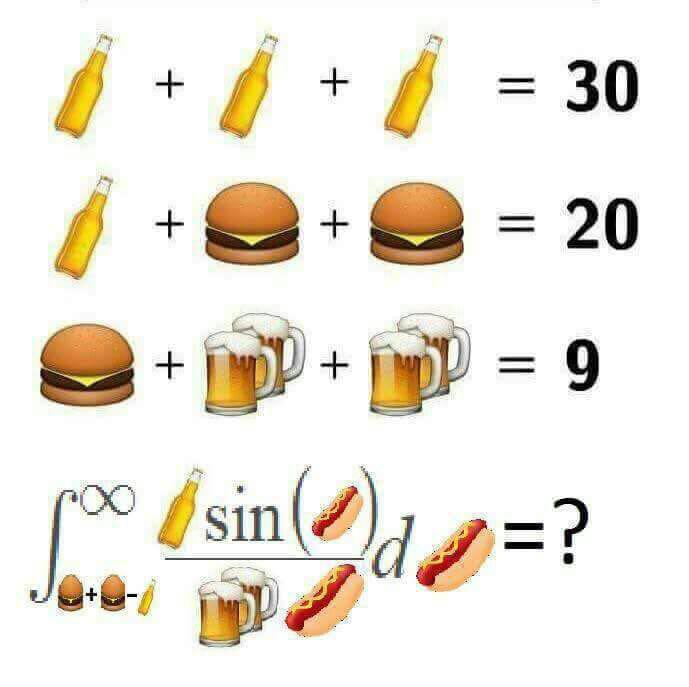
The beginning of the meme is, as you most probably know, those famous “linear system of equations with emojis as variable names”. Fun fact about those memes: Most of them present systems that are quasi triangular, and hence, very very easy to solve. Anyways… In general, they give you 3 or 4 equations with right hand side values and asks you to compute a 4th or 5th equation that is, in general, another linear combination of the variables.

In the case of the meme in this post, the 4th equation is nothing like a linear combination of the values. In fact, it is some weird looking complex mathematical aberration! This is where the meme ends for most people. The funny in that meme is that nobody can solve it. So, you suppose to send that to your annoying friend that keeps sending regular linear combination meme to you, solving all of them to show that he is a math genius.

Well… It turns out that the 4th equation is solvable! Moreover, it involves the integral of a very famous function (sinc(x)) which has a very elegant solution for when the limits involves infinity and zero. And yet more, that is despite the fact that it have no closed form solution for arbitrary limits.

Translating the emojis to variables, we arrive at the following integral

    \[\int\limits_{2b - a}^\infty {\frac{{a\sin (x)}}{{c\,x}}{\text{d}}x}\]

which, with numbers (solving the trivial linear system of emojis), becomes

    \[5\int\limits_0^\infty {\frac{{\sin (x)}}{x}{\text{d}}x}\]

The goal of this post them, is to solve this integral using almost all steps as purely algebraic manipulations. We are also going to use rules of calculus too, obviously. Moreover, we will have to go though some complex numbers too (yes, we go that deep in a innocent internet meme). Lets now solve the sinc function integral.

We start by acknowledging that sin(x)/x is a function with an anti-derivative that is very hard to find. If only we had something to relate easier functions to integrate (like exponentials)… But wait, we have! The first exponential that we can exchange, is the sine function (as you probably already guessed). So lets use Euler’s identity:

    \[\sin (s) = \frac{{{e^{sj}} - {e^{ - sj}}}}{{2j}}\]

Although this is not a pure algebraic step, I will save the Taylor expansion prove of this one, for it is a very, very known relation.

The second one is the “jump of the cat” as we say in my country. I found that in an awesome StackExchange answer[1]. It has to do with the Laplace transform of the step function $u(t)$. In short, it states that the Laplace of the step function is the inverse function. The relation has a simple one line of proof as

    \[L\left\{ {u(t)} \right\} = \int\limits_0^\infty {{e^{ - st}}{\text{d}}t} = \left. {\frac{{{e^{ - st}} - {e^{ - st}}}}{{ - s}}} \right|_0^\infty = \frac{1}{s}\]

Hence

    \[\int\limits_0^\infty {{e^{ - st}}{\text{d}}t} = \frac{1}{s}\]

Holly butter ball! Now we can relate the inverse function with the exponential 😱. I wish I could use emojis on technical papers. They convey so much of the authors wonder when some beautiful math trick is used… %-)

Now lets change the names of the variables to

    \[\int\limits_0^\infty {\frac{{\sin (s)}}{s}{\text{d}}s}\]

which makes our original sinc integral to become

    \[\int\limits_0^\infty {\int\limits_0^\infty {{e^{ - st}}\frac{{{e^{sj}} - {e^{ - sj}}}}{{2j}}{\text{d}}t} {\text{d}}s}\]

Ok, the s integral is easy and here are the steps to solve it (it’s an exponential integral)

    \[\begin{gathered} \frac{1}{{2j}}\int\limits_0^\infty {\int\limits_0^\infty {{e^{ - s(t - j)}} - {e^{ - s(t + j)}}{\text{d}}s} {\text{d}}t} \\ \frac{1}{{2j}}\int\limits_0^\infty {\left. { - \frac{{{e^{ - s(t - j)}}}}{{t - j}} + \frac{{{e^{ - s(t + j)}}}}{{t + j}}} \right|_0^\infty {\text{d}}t} \\ \frac{1}{{2j}}\int\limits_0^\infty { - \frac{{{e^{ - \infty (t - j)}}}}{{t - j}} - \frac{{{e^{ - \infty (t + j)}}}}{{t + j}} + \frac{{{e^{ - 0(t - j)}}}}{{t - j}} - \frac{{{e^{ - 0(t + j)}}}}{{t + j}}{\text{d}}t} \\ \frac{1}{{2j}}\int\limits_0^\infty {\frac{1}{{t - j}} - \frac{1}{{t + j}}{\text{d}}t} \end{gathered}\]

Now we have a integral in t. This one is a lot of fun. Lets start by separating the two integrals as

    \[\begin{gathered} \frac{1}{{2j}}\int\limits_0^\infty {\frac{1}{{t - j}}{\text{d}}t} - \frac{1}{{2j}}\int\limits_0^\infty {\frac{1}{{t + j}}{\text{d}}t}\end{gathered}\]

and do the following substitution.

    \[\begin{gathered} {u_1} = t - j \\d{u_1} = dt \\{u_2} = t + j \\ d{u_2} = dt \end{gathered}\]

Now we foresee that we are going to have some trouble with this infinity and the logs that will appear… Hum… Lets be careful (to not say formal) and wrap that around a limit. We deal with the limit later. Ok, so we have the following inverse integral (with is also simple to solve inside the limit)

    \[\begin{gathered} \frac{1}{{2j}}\mathop {\lim }\limits_{a \to \infty } \int\limits_{ - j}^{a - j} {\frac{1}{{{u_1}}}{\text{d}}{u_1}} - \int\limits_j^{a + j} {\frac{1}{{{u_2}}}{\text{d}}{u_2}} \\ \frac{1}{{2j}}\mathop {\lim }\limits_{a \to \infty } \left. {\ln ({u_1})} \right|_{ - j}^{a - j} - \left. {\ln ({u_1})} \right|_j^{a + j} \\ \frac{1}{{2j}}\mathop {\lim }\limits_{a \to \infty } \ln (a - j) - \ln ( - j) - \ln (a + j) + \ln (j) \\ \frac{1}{{2j}}\mathop {\lim }\limits_{a \to \infty } \ln (a - j) - \ln (a + j) + \ln (j) - \ln ( - j) \\ \frac{1}{{2j}}\mathop {\lim }\limits_{a \to \infty } \ln (a - j) - \ln (a + j) + \ln \left( {\frac{j}{{ - j}}} \right) \\ \frac{{\ln \left( { - 1} \right)}}{{2j}} + \frac{1}{{2j}}\mathop {\lim }\limits_{a \to \infty } \ln (a - j) - \ln (a + j) \end{gathered}\]

The lets now investigate this peculiar limit (and begin the fun part). We start by acknowledging that it is a complex-number limit, hence not straight forward to evaluate. Then, we do the second “jump of the cat” (small when compared with the Laplace one before) and change the limit of infinity with a limit to zero as

    \[\begin{gathered} \mathop {\lim }\limits_{a \to \infty } \ln (a - j) - \ln (a + j) \hfill \\ \mathop {\lim }\limits_{a \to 0} \ln \left( {\frac{1}{a} - j} \right) - \ln \left( {\frac{1}{a} + j} \right) \end{gathered}\]

Now we just do some algebraic steps as follows

    \[\begin{gathered}\mathop {\lim }\limits_{a \to 0} \ln \left( {\frac{{1 - aj}}{a}} \right) - \ln \left( {\frac{{1 + aj}}{a}} \right) \hfill \\\mathop {\lim }\limits_{a \to 0} \ln \left( {1 - aj} \right) - \ln (a) - \ln \left( {1 + aj} \right) + \ln (a) \hfill \\\mathop {\lim }\limits_{a \to 0} \ln \left( {1 - aj} \right) - \ln \left( {1 + aj} \right)\end{gathered}\]

And voilá! Put a=0 and we have out first part of the integral

    \[\ln \left( 1 \right) - \ln \left( 1 \right) = 0\]

Now we have to figure what the heck is this negative logarithm thing…

    \[\frac{{\ln \left( { - 1} \right)}}{{2j}}\]

To do that, lets make the cat jump again and write -1 as e^{j\,\pi}

now we have

    \[\frac{{\ln \left( { - 1} \right)}}{{2j}} = \frac{{\ln \left( {{e^{j\pi }}} \right)}}{{2j}}\]

which is just \frac{\pi }{2} . %-)

Finally coming back to the original integral we have

    \[5\int\limits_0^\infty {\frac{{\sin (x)}}{x}{\text{d}}x} = \frac{5\,\pi}{2}\]

Which is the actual answer for the meme %-).

Conclusion

So, if you see this meme on your WhatsApp, feel free to answer in a very “disdain” way: “Humpf! This is trivially equal to \frac{5\,\pi }{2} !”. If they doubt you, share this post with them %-).

I hope you like this post! Feel free to share it or use any of this derivations on your own proofs! See you in the next post!

References:

[1] – Stack Exchange Mathematics – Integration of Sinc function

Leave a Comment